The usually excellent Marginal Revolution blog features daily “Assorted Links” that point to interesting articles on an exceptionally wide variety of topics, most often related to economics or sociology. Monday’s included a truly awful article from “engadget.com” on “Rain as a source of renewable energy”. Of course, there are many awful things on the internet, and on the overall scale of awfulness this isn’t anywhere near the top. I thought it worth commenting on, however, because it’s related to issues of energy and the environment, physics, and scientific literacy, all topics I care about. (Also, I thought I’d see if I could write a post in less than an hour. The answer: no. Not even close.)
The article notes that researchers have harnessed falling rain to generate electricity, lighting up LEDs.
There is nothing at all surprising about this in principle One of the most basic tenets of physics is that energy can be converted from one form to another. Here, the kinetic energy of a raindrop is transformed into electrical energy. A wind turbine transforms the kinetic energy of air into electrical energy. I could lodge piezoelectric crystals in my throat and generate electricity each time I swallow. The concept is straightforward.
The question to ask — in fact, the only question to ask — is how much power can be generated by this transformation. Stunningly, the article doesn’t bother to ask this question. The only number that appears is in the statement that “a single drop can muster 140V.” This is like saying my mass is two meters — nonsense. Volts are a measure of voltage, not power. One can have millions of volts and hardly any power, for example, if that voltage drives a very small current. What matters for generating power is, in fact, power. Anyone who doesn’t understand that volts don’t measure power has no business writing about renewable energy, or technology of any sort.
How much power can we expect from rainfall? Let’s estimate it! (If you don’t care about how to estimate it and just want the answer, skip to the last paragraph of the next section.)
It’s immensely powerful and liberating to perform back-of-the envelope estimates of all sorts of quantities. We work on this a lot in my physics classes for non-science majors — more on that in a moment.
As noted, the basic idea is simple: a falling rain drop has some kinetic energy; we convert that into electrical energy. For the purpose of estimating the power captured, it doesn’t matter at all how this conversion happens. (In the research paper the article is about, as I’ll note below, it’s through a triboelectric mechanism.) It only matters how much kinetic energy per time interval is converted — the universe dictates that you can’t get more output than this, no matter what you do.
Let’s be charitable and assume we capture all the kinetic energy — the drop doesn’t bounce, and our conversion device is perfect. (This is, of course, completely unrealistic — I’d be surprised if we could keep even 10% of the energy, but let’s set an upperbound on power from rainfall.)

The mass of a raindrop and its velocity give its kinetic energy. The rate at which energy is transferred is the power — that’s the definition of power. In equations:
K = (1/2) M v2,
where K is the kinetic energy, M is the drop’s mass, and v is its velocity. The power
P = K/t.
What is t? If we take t to be the time the drop spends splattering, we’ll get the instantaneous power. This is some large number while there’s a raindrop hitting the surface, and zero in the interval between drops. What matters is the average power, i.e. K/t where t is the time between drops, i.e. the time interval per which we get K amount of energy. (It’s like having car that goes at 100 miles per hour that runs for a few seconds and then must pause for a few minutes. The relevant speed for getting somewhere isn’t 100 miles per hour, but rather the average distance per time.)
What are all these numbers? A falling raindrop reaches a terminal velocity due to air resistance. We can be lazy and look it up, or we can estimate it (see Note [1]). In either case, it depends on the droplet size, and is about 1 to 10 meters per second (2 – 20 miles per hour). Again let’s be charitable and say v = 10 m/s, taking the upper end.
What’s M? We could figure out the mass of one raindrop and then multiply our estimate of the power per drop by the number of raindrops hitting when it rains, but as is often the case in physics there are simpler ways to think about the problem. Rainfall is often measured as the depth of water received over some amount of time, for example 10 millimeters per hour. That’s telling us that the total of all the droplets is equivalent to a 10 millimeter thick slab arriving once per hour.
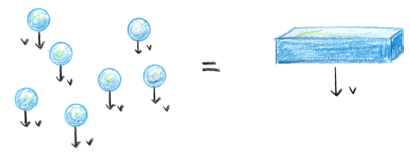
Therefore, all we need to do is calculate the kinetic energy of the falling 10 millimeter thick slab, divide by one hour, and we’ve got our power. That kinetic energy is
K = (1/2) × the mass of the slab × v2,
or in other words
K = (1/2) × the density of water × the volume of the slab × v2.
The volume of the slab is its height times its area. The height of the slab divided by the time per slab is simply the rainfall rate, which we’ll denote as Q (0.01 m/hour in the example). Writing the density of water as ρ and the area of the slab as A, the power is
P = K/t = (1/2) ρ A Q v2,
so the power per unit area of rainfall we collect is
P/A = K/t = (1/2) ρ Q v2.
A moderate rainfall (according to Wikipedia) is about 10 mm/hour, or 3 × 10-6 meters per second. The density of water is about 1000 kg/m3, and v, as we’ve said, is about 10 m/s.
Plugging it all in, the power per unit area we’d expect to collect is at best, with perfect efficiency, about 0.1 Watts per square meter.
My explanation here has been fairly terse. See Note [2] for a comment on how I’d go about this in a class.
A tenth of a Watt per square meter is pathetically low, and keep in mind that we’ve been generous in multiple places. I’d expect less than a tenth of this, i.e. a few milliWatts per square meter, in any real implementation.
For a sense of scale, a solar panel gets around ten thousand times this, or 100 Watts per square meter. A monkey turning a hand-crank generator would generate about 100 Watts, and you could fit several monkeys in a square meter.
What does the actual research paper, rather than the popular press articles, say? It is:
W. Xu, H. Zheng, Y. Liu, X. Zhou, C. Zhang, Y. Song, X. Deng, M. Leung, Z. Yang, R. X. Xu, Z. L. Wang, X. C. Zeng, Z. Wang, “A droplet-based electricity generator with high instantaneous power density.” Nature, 1-5 (2020). https://doi.org/10.1038/s41586-020-1985-6
The article describes a clever and interesting energy conversion mechanism. Essentially, the falling drop’s kinetic energy is converted to electrical energy via triboelectricity — rubbing things together generates charges at the surfaces, and these charges end up forming an electric current as the water droplet bridges electrodes. At least I’m pretty sure of this — the paper is quite unclear, and for a while I was wondering if any electricity is generated at all or if the water simply serves to complete an already existing switch.
Shockingly, and inexcusably in my opinion, the researchers only state the instantaneous power (50 W/m2) of their device — the peak, as droplets hit — rather like the “100 mph” in our car example above. 50 W/m2 sounds impressive, but it’s very misleading.
When it rains, what fraction of the space is taken up by water droplets, rather than air? (In class, this would be a good “clicker question” followed by an estimation exercise.) Again, we already have the tools in hand. The rainfall rate is Q (e.g. 10 mm/hr, or 3 × 10-6 meters per second). The speed of the drops is v = 10 m/s. If all the space were filled with water, Q would be the same as v. It’s not — it’s about 107 times smaller. About 1/10,000,000th of the volume around is is filled with water when it’s raining. That’s enough to get us wet, but walking through the rain isn’t like walking through a swimming pool!
Should anyone care about the researchers’ rain harvesting? The relevant number is 50 W/m2 divided by 107, or about 10-5 W/m2. In other words, 0.01 mW/m2, just like we estimated. You can’t cheat physics!
(There is, by the way, a very good way to harness rain for renewable electricity: let geography concentrate the water into a river, then build a dam and a hydroelectric plant!)
The combination of poor writing and fundamental unimportance leaves me very surprised that the paper got into Nature, one of the most prestigious scientific journals. Nature is extremely difficult to get published in; who knows what the editors and reviewers were thinking…
There is no conceivable way that the power captured from rainfall, even if 100% efficient, could possibly compensate for the cost (in money or energy) of building the power harvesting apparatus. There’s also no conceivable way that pursuing this is a better use of time and resources than other (very good) paths to renewable energy.
One might say “it’s better than nothing,” but no, it isn’t. As David MacKay eloquently wrote in the excellent “Sustainable Energy Without the Hot Air,”
Don’t be distracted by the myth that “every little helps.” If everyone does a little, we’ll achieve only a little.
Scale actually matters.
The main reason I’m upset by all this is that it highlights failures of journalism and of education. There are more poor pop-science articles about this rain energy paper than the one I cited at the top of the post, e.g. in Popular Mechanics. (I think Popular Mechanics used to be a legitimate magazine). Articles like this one are very common. One would hope that their authors, rather than swallowing uncritically university press statements (often awful in themselves, and basically just advertising), would think, and ask questions. The key question to ask if looking for an announcement like this, about power generation, is how much power can be generated. The fact that people writing about science or technology don’t even know to ask such questions is dispiriting.
More generally, pop-science articles rarely ask any quantitative questions, as if numbers were tangential to understanding the meaning or importance of scientific news. There are exceptions, of course — The Economist is usually good, and there are several very good video sources of science popularizations (e.g. the Veritasium channel) — but the general state of things is poor.
Why? We can lament the general collapse of journalism, but I think a broader culprit is that scientific literacy and quantitative reasoning are still not seen as integral to the makeup of a “well-educated” person. This isn’t just unfortunate in itself, but has consequences for how, for example, we deal broadly with topics related to energy, population, and more. Coincidentally, on the same day I read the awful rain power article, I attended a meeting about how to continue reforming the University of Oregon’s general education requirements. Improved quantitative literacy was high on several people’s list of wishes, which is encouraging. How to implement this remains to be seen.
A quickly drawn splash.
— Raghuveer Parthasarathy, February 12, 2020
[1] Interestingly, figuring out the terminal velocity of a raindrop is essentially the same problem as figuring out the power needed to move a car at constant, high velocity — something we do in my Physics of Energy and the Environment course for non-science majors. I’ve spent too long on this post as it is, so I’ll leave this as an exercise for the reader. In essence, the thing to figure out is the power needed to “push” a column of air, raising its kinetic energy from zero to some steady value. Here, the raindrop pushes the air; on a highway, it’s the car. Here’s a link to a paper in which I describe this more.
[2] My explanation of our order-of-magnitude estimate here is rather terse. Note, however, that it’s based on just two very basic equations (an equation for Kinetic Energy, and an equation relating power, energy, and time) — the rest is all just reasoning and estimation. We spend a lot of time on these sorts of exercises in my classes for non-science-major undergraduates, especially “Physics of Energy and the Environment,” which I’ve written about a few times. There, I guide students more slowly, often making use of small-group exercises structured around worksheets interspersed with questions, answers, and mini-lectures. Here’s an example of a worksheet, part of an activity that extends over two class periods in which we figure out how much power is needed to drive a car on a highway. (In other words, is it possible to make a 10 horsepower car, or is there a minimum power requirement set by the underlying physics?) That exercise is similar in many ways to the raindrop analysis, requiring just one or two basic concepts, some drawing, and paring down problems to their essential components; see the paper in Note [1] for details.